
![]() No aqueous solution is ideal
No aqueous solution is ideal
![]() D2O
and T2O differ significantly from H2O
in their physical properties
D2O
and T2O differ significantly from H2O
in their physical properties
![]() Liquid H2O
and D2O differ significantly in their phase behavior
Liquid H2O
and D2O differ significantly in their phase behavior
![]() H2O
and D 2O ices differ significantly in their quantum behavior
H2O
and D 2O ices differ significantly in their quantum behavior
![]() The mean kinetic energy of water's hydrogen atoms increases at low temperature
The mean kinetic energy of water's hydrogen atoms increases at low temperature
![]() Solutes have varying effects on properties
such as density and viscosity
Solutes have varying effects on properties
such as density and viscosity
![]() The solubilities of nonpolar gases in
water decrease with temperature to a minimum and then rise
The solubilities of nonpolar gases in
water decrease with temperature to a minimum and then rise
![]() The dielectric
constant of water and ice are high
The dielectric
constant of water and ice are high
![]() The dielectric
constant shows a temperature maximum
The dielectric
constant shows a temperature maximum
![]() The relative permittivity shows a 'kink' in its behavior with the temperature at 60 °C
The relative permittivity shows a 'kink' in its behavior with the temperature at 60 °C
![]() The imaginary part of the dielectric constant shows a minimum near 20 K
The imaginary part of the dielectric constant shows a minimum near 20 K
![]() Proton and hydroxide ion mobilities are
anomalously fast in an electric field
Proton and hydroxide ion mobilities are
anomalously fast in an electric field
![]() The electrical conductivity of water
increases to a maximum at about 230 °C
The electrical conductivity of water
increases to a maximum at about 230 °C
![]() The electrical conductivity of water
rises considerably with frequency
The electrical conductivity of water
rises considerably with frequency
![]() Acidity constants of weak acids show temperature minima
Acidity constants of weak acids show temperature minima
![]() X-ray diffraction shows an unusually
detailed structure
X-ray diffraction shows an unusually
detailed structure
![]() Under high pressure, water molecules move
further away from each other with increasing pressure
Under high pressure, water molecules move
further away from each other with increasing pressure
![]() Water adsorption may cause negative electrical resistance
Water adsorption may cause negative electrical resistance
Ideality depends on the structure of the solvent being unaffected by the solute. Water is not even close to being a homogeneous phase at the molecular level. Local clustering will be affected by the presence of solutes, so changing the nature of the water. Even solutions of HDO in H2O do not behave ideally. j Although most non-aqueous solutions also show deviations from ideality at higher concentrations, the deviations that occur in aqueous solutions are generally much more extensive. However, close to ideality in aqueous solutions can be achieved if the 'free' water is considered when some of the water molecules are bound more strongly to the solutes than they are to other 'free' water molecules [3505].
[![]() Anomalies page: Back to Top
Anomalies page: Back to Top ![]() ]
]
Different isotopic forms of compounds usually behave very similarly to each other. However, nuclear quantum effects in the water molecule are significant and differ between the isotopic forms. The 50% lighter mass of hydrogen compared with deuteron cause the properties of light water H2O to be more influenced by nuclear quantum effects than those of heavy water D2O. The heavier forms of water (D2O where D = deuterium, 2.0141 g ˣ mol−1; and T 2O where T = tritium, 3.0160 g ˣ mol−1) form stronger hydrogen bonds than light water (H2O where H = protium, 1.0078 g mol−1) and vibrate less. Hence, they are more ordered than ordinary water, as shown by their greater molar volumes, o are more tetrahedral and have more hydrogen bonds [1485]. This causes many of their properties (such as the viscosity, self-diffusion coefficient, protein solubility, protein stability [3562], toxicity a and biological activity [2265], including the effect on the frequency of circadian oscillations [1395]) to be different from those expected from a simple consideration of their increased mass (for example, the D2O/H2O viscosity ratio rises from about 1.16 at 100 °C to around 2.0 in deeply supercooled water [23b]. This difference appears as a shift in the equilibrium position equivalent to a slight increase in temperature [425]; for example, viscosity data has been reconciled if the temperatures are shifted by 6.498 °C and 8.766 °C for D2O and T2O, respectively [73]. b H2O is about four-fold stronger as an acid than D2O at 25 °C, and H3O+ in H2O is 1.5 times as strong an acid as D3O+ in D2O. Remarkably, the difference in the specific heat minimum between H2O and D2O is over 80 °C. Most of the differences between the behavior of H2O and D2O has been explained as due to the nuclear quantum effects i inherent in the large mass difference between the hydrogen and oxygen atoms [554. However, it has subsequently been found find that liquid D2O is more structured than liquid H2O. This work predicts the intra- and intermolecular structures of liquid T2O, HDO and HTO [4448]. Although the electron densities of the different isotopic forms of liquid water have proved, so far, to be indistinguishable [566], it is expected that the O-D bond length is shorter than that of O-H due to its smaller asymmetric vibration and the smaller Bohr radius of D relative to H. This gives rise to small differences in the size and direction of the dipole moment between HDO and H 2O [1174], which further confuses any analysis of the structure of water containing mixed hydrogen isotopes.
Even though the amount of deuterium in commonly-found water is low (≈ 16 mM), the properties of such water are different to water containing protium (1H) only (deuterium-depleted water) [2063,3164]; as example, the proton spin-spin relaxation (T2) is 0.347 ± 0.024 s for deuterium depleted water (D/H = 3 ppm) compared with 2.000 ± 0.140 s for 'normal' water (D/H = 140 ppm), and the diffusion coefficient is 37% greater in deuterium-depleted water) [3164].
Almost pure H2O and D2O exist, m but HDO can never be more than about 50% pure, existing only in the presence of both H2O and D2O. Mixtures of H2O and D2O rapidly equilibrate (via proton transfer by self-dissociation and recombination) to form HDO:
H2O
+ D2O ![]() 2HDO
Keq = 3.86, 25 °C [609], ΔHmix = 129.4 J mol−1 [654]
2HDO
Keq = 3.86, 25 °C [609], ΔHmix = 129.4 J mol−1 [654]
Mole fractions of the H2O/D2O mixtures
The dotted lines show the original amounts
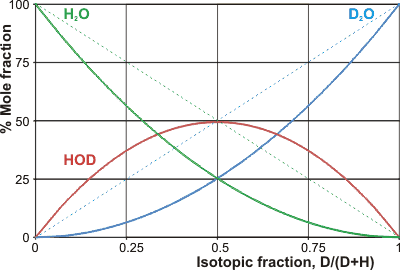
which is close to a total randomization of the hydrogen atoms (that is, equal concentrations of HOH, HOD, DOH and DOD that would be Keq = 4) but is reflected in a slight preference for the partitioning of the deuterium-containing species into the more extensive and stronger hydrogen-bonded clusters. l ΔHmix is the enthalpy of mixing, which may be considered high. Thus a mixture of 20% D2O and 80% H2O (by volume and by mol; ≈ 11 mol D2O per liter solution) gives a mixture of 64% H2O, 32% HDO, and 4% D2O (by mol, ≈ 36 M H2O, ≈ 2 M D2O and ≈ 18 M HDO) and lower the temperature of the mixture by over 0.5 °C (if no heat flows in from the environment). The Keq decreases with decreased temperature [126a] and increased hydrogen-bond cooperativity [985]. Even the properties of HDO deviate from those expected from a consideration of the properties of H2O and D2O [126b], with the D-atom preferring to be hydrogen-bonded over the H-atom except where the hydrogen-bond is particularly short (as in H5O2+) [985]. Surprisingly, D2O does not mix as readily with H2O as might be pre-supposed [1472], presumably as the preferred D2O clustering holds the D2O molecules together. This tendency gives anomalous cooling curves in unstirred D2O/H2O mixtures [1472], slow equilibration, and unexpected entropic effects [1855]. Mixing 1:1 H2O and D2O gives rise to a small heat of mixing (≈ 1.3 kJ ˣ mol−1).
The vibrational spectrum of HDO is fundamentally different from either H2O or D2O due to the separation of the two hydroxyl (O-H and O-D) vibrations in HDO but their combined motion in H2O and D2O. In HDO, the H atom is more reactive and more easily dissociated than the D atom. As hydrogen-bonding is a property of at least two water molecules, isotopic mixtures contain many differently paired (and more extensive) species, each of which may present different properties to those in natural liquid water. Care must be taken over extrapolating the properties of H2O/D2O mixtures (often used in neutron scattering and vibrational spectroscopic studies) to those of normal liquid water (that is, 99.97% H2O). For example, D2O is preferentially found at hydrophilic interfaces [1342].
Liquid T2O is corrosive due to self-radiolysis (
Even H218O behaves differently from H216O due to reduced quantum translational motions, reducing the size of the first shell local hydrogen-bonded tetrahedron but leaving the non-bonded water distances almost the same [1035]. Although D2O has a similar mass (only 0.04% heavier than H218O), its behavior is much more affected by the isotopic substitution due to the altered mass distribution influencing its librations and hence the local environment of both the first and second aqueous shells [1035]. H217O and HDO behave as different molecules to H216O in mixtures with H216O as shown by their colligative properties, the freezing points rising with the molality of H217O or HDO (freezing points rising here as they form mixed solid solutions) [1470].
At 50 °C, the vapor pressure of H2O is 10% greater than D2O, so the concentration of deuterium in the liquid phase of water increases on evaporation. However, at about 220 °C both vapor pressures are equal and between 220 °C, and its critical point, D2O has a higher vapor pressure than H2O. Both light (H2O) and heavy water (D2O) are used as moderators in nuclear reactors; reducing the speed of fast neutrons and turning them into thermal neutrons capable of sustaining a nuclear chain reaction. Moderation is more rapid using light water but more efficient using heavy water, as light water scatters and absorbs significantly greater than heavy water. Although for many years it was thought that light (H2O) and heavy water (D2O) apparently could not be distinguished by taste, it has been found that heavy water elicits a sweet taste, mediated by the human Taste Receptor type 1 member 2/Taste receptor type 1 member 3 (TAS1R2/TAS1R3, sweetness receptor) taste receptor [3989]. Large amounts of heavy water may be toxic due to reaction kinetic effects [3567].
[![]() Anomalies page: Back to Top
Anomalies page: Back to Top ![]() ]
]
The phase behavior of liquid H2O and D2O differ, with the triple point of D2O being 3.79 °C and 48.439 Pa higher than that of H2O, their vapor pressure curves crossing at 221 °C, and the critical point of D2O being 3.249 °C and 393 kPa lower [1007]. This isotope effect originates in the reduced zero-point vibration p of D2O that reduces its van der Waals volume (by about 1%) and its associated repulsive effect within the hydrogen bonds at lower temperatures, increasing the D2O-D2O hydrogen-bond strength. c At higher temperatures, the transition to the excited state is more easily accomplished in D2O (≈ 2450 cm−1, relative to H2O ≈ 3280 cm−1). Due to the asymmetry of the vibration, this increases D2O's effective van der Waals volume and reverses the relative repulsive effect, reducing the D2O-D2O hydrogen bond strength at higher temperatures. d
The miscibility properties of liquid H2O and D2O also differ, with 3-methylpyridine being always miscible in liquid H2O but immiscible in liquid D2O between 38.5 °C and 117 °C at normal pressure [1976].
[![]() Anomalies page: Back to Top
Anomalies page: Back to Top ![]() ]
]
The volume isotope anomaly for ices, from [2936]
![the volume isotope anomaly for ices, from [2936] the volume isotope anomaly for ices, from [2936]](images/viap.gif)
Replacing hydrogen (1H) by deuterium (2H) results in a greater volume (≈ 0.1%) of hexagonal ice (the volume isotope anomaly; the nuclear isotope effect). Thus, H2O has a larger molar density (50.899 mol ˣ kg−1) than D2O (50.816 mol ˣ kg−1) at their melting points. This is in contrast to the expected behavior (e.g., solid 20Ne is 0.6% more voluminous than 22Ne) due to the expected greater nuclear motion of lighter isotopes [1819]. Furthermore, the anomalous effect in water increases with temperature, even though a typical isotope shift should decrease with temperature [1819]. This anomalous behavior is due to the significantly greater covalent O-H asymmetric vibration and the O-H distance being longer than the O-D distance. Also, the hydrogen-bond donor-acceptor (oxygen–oxygen; H-O-H····OH2) distance changes upon isotopic substitution (the Ubbelöhde effect also called the secondary isotope effect) [3528].
A similar anomaly occurs with ice VIII under pressure (see right) but not with the ionic ice X [2936]. Ices Ic, II, III, V, IX, XII, XIII, and XIV are also likely to have this volume isotope anomaly [2936, 3152]. Unexpectedly, while the ice II to ice Ic transition is endothermic for H2O (+40 J ˣ mol−1), it is exothermic for D2O (−140 J ˣ mol−1) [4132].
As the Keq decreases with decreased temperature [126a] and increased hydrogen-bond cooperativity [985] (see above), at temperatures close to 0 K, this may mean that H2O and D2O may form separate phases and are no longer in equilibrium [985].
[![]() Anomalies page: Back to Top
Anomalies page: Back to Top ![]() ]
]
The mean kinetic energy of liquid water's hydrogen atoms has been reported to reach a maximum at about 7 °C, then reducing on cooling to a minimum value at about 0 °C [1596]. However, it should be noted that a simulation does not show this anomaly [1760]. Also, new experimental results, benchmarked with those obtained for ice [2768], do not show this anomaly. At the current time, this anomaly should be considered suspect.
[![]() Anomalies page: Back to Top
Anomalies page: Back to Top ![]() ]
]
Solutes will interfere with the cluster equilibrium by favoring either open or collapsed structures. Any effect will cause the physical properties of the solution, such as density or viscosity, to change. Solutes have a lower than expected impact on both the cryoscopic constant (that is, an effect of solute on freezing point depression, 1.86 K kg mol−1, compare CCl4 30 K kg mol−1) and ebullioscopic constant due to water's low molar mass and high heats of fusion and evaporation respectively.
[![]() Anomalies page: Back to Top
Anomalies page: Back to Top ![]() ]
]
nonpolar gases are poorly soluble in water. Most gaseous solutes dissolve more in most solvents as the temperature is raised. However, nonpolar gases are much more soluble in water at low temperatures than would be expected from their solubility behavior at high temperatures.
Solubilities for the noble gases, from [IAPWS, 1166]

The solubilities of the noble gases are shown opposite [IAPWS, 1166] and are tabulated below. Their hydration may
be considered as the sum of two processes: (A) the endothermic opening
of a clathrate pocket in the water and (B) the exothermic placement
of a molecule in that pocket, due to the multiple van der Waals dispersion interactions (for example, krypton dissolved in water is surrounded by a clathrate cage with 20 Kr···OH2 such interactions [1357]). In water at low temperatures, the energy required
by process (A) is minimal as such pockets may be easily formed
within the water clustering (by CS ![]() ES).f Using the noble gases to investigate the solvation of nonpolar gases is helpful as they are spherically symmetrical and have low polarizability. In contrast, the shape and polarizability may confuse the hydration of other gases.
The solubility of the noble gases increases considerably as the temperature is lowered. Their enthalpy and entropy of hydration become more negative as their fit into the water dodecahedral clathrate improves.
ES).f Using the noble gases to investigate the solvation of nonpolar gases is helpful as they are spherically symmetrical and have low polarizability. In contrast, the shape and polarizability may confuse the hydration of other gases.
The solubility of the noble gases increases considerably as the temperature is lowered. Their enthalpy and entropy of hydration become more negative as their fit into the water dodecahedral clathrate improves.
Property |
He |
Ne |
Ar |
Kr |
Xe |
Rn |
|
|---|---|---|---|---|---|---|---|
Atomic number |
2 |
10 |
18 |
36 |
54 |
86 |
|
1.08 |
1.21 |
1.64 |
1.78 |
1.96 |
2.11 |
||
Partial molar volume in H2O [3763], cm3 ˣ mol−1 |
24.6 | 24.6 | 31.5 | 37.4 | 45.0 | 49.4 | |
ΔG° of solution in H2O at 25 °C, kJ ˣ mol−1 [1296] |
29.41 |
29.03 |
26.25 |
24.80 |
23.42 |
19.29 * | |
ΔH° of solution in H2O at 25 °C, kJ ˣ mol−1 [1296] |
-0.59 |
-3.80 |
-11.98 |
-15.29 |
-18.99 |
-21.37 * | |
ΔS° of solution in H2O at 25 °C, J ˣ mol−1 ˣ K−1 [1296] |
-100.6 |
-110.1 |
-128.2 |
-134.5 |
-142.2 |
-144.0 * | |
ΔCP of solution in H2O at 25 °C, J ˣ mol−1 ˣ K−1 [678] |
105.2 | 150.8 |
178.3 |
204.4 | 197.5 |
292.8 | |
Solubility, mM, 5 °C, 101,325 Pa [1166] |
H2O |
0.41 |
0.53 |
2.11 |
4.20 |
8.21 |
18.83 |
D2O |
0.49 |
0.61 |
2.38 |
4.61 |
8.91 |
20.41 |
|
H2O |
30 |
50 |
90 |
108 |
110 |
91 |
|
D2O |
53 |
53 |
98 |
108 |
116 |
||
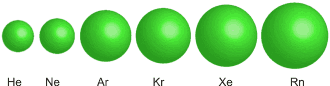
Oxygen (O2) and nitrogen (N2) molecules behave similarly (solubility minima of N2 at 74 °C and O2 at 94 °C, IAPWS), although their solubilities are low (O2, 1.92 mM in H2O, 2.14 mM in D2O; N2, 0.94 in H2O, 1.05 mM in D2O; all at 5 °C, 101,325 Pa [1168]). The higher solubility of O2 over N2, despite its lesser clathrate forming ability [1168] has been proposed due to its formation of weak hydrogen bonds to water [1168].g
Therefore, the solubilization process is exothermic (that is, has negative ΔH) and (as predicted by Le Chatelier's principle) solubility decreases with temperature rise. At high temperatures (often requiring high pressure), the natural clustering is much reduced, causing greater energy to be required for opening the pocket in the water. The solubilization process becomes endothermic, and (as predicted by Le Chatelier's principle) solubility goes through a minimum before increasing with temperature rise (being fully miscible under supercritical conditions).
Henry's constants, 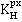 , for the noble gases
, for the noble gases
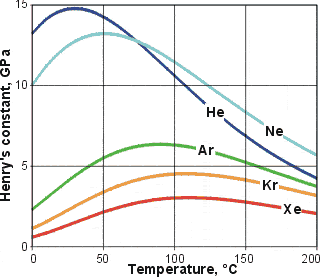
The more attractive the solute-water van der Waals dispersion interactions
(due both to atomic number dependence and goodness of
fit within the clathrate pocket), the greater the inherent
exothermic nature of the process and therefore, the higher
the temperature minimum (see Table above) and
the greater the temperature range of negative temperature
solubility coefficient. Similarly, Henry's constants (![]() =
partial pressure/mole fraction; represents volatility, see opposite)
exhibit increasing maxima with increasing size (the maxima are the same as the solubility minima above).
=
partial pressure/mole fraction; represents volatility, see opposite)
exhibit increasing maxima with increasing size (the maxima are the same as the solubility minima above).
The poor solubility of nonpolar gases in water, despite their negative enthalpy change on dissolution, is due to the positive free energy changes (+ve ΔG) attributed to the anomalously large negative entropy changes (-ve TΔS). These entropy changes are caused by the structural enhancement of the water (ES) clusters. This conclusion is reinforced by the enhanced heat capacity (ΔCP) of these solutions (+ve Cp, characteristic of a decrease in the degrees of freedom of the water solvent). This structural enhancement may include fixing the cluster centers, preventing the randomizing flickering between clusters otherwise evident, and ordering the inner dodecahedral water shells surrounding the solute molecules. There is also a decrease in volume (-ve ΔV) showing a reduction in the unoccupied space within the water solvent and also indicative of the gases occupying the pre-existing, if collapsed, clathrate sites.
Very large hydrophobic molecules and structures, such as membranes and surfactants (and oils and large hydrocarbons) are not soluble in water, which allows for the organelles that we need to make up our cells.
Counterintuitively despite forming stronger hydrogen bonds, D2O is a better solvent than H2O for nonpolar gases, it is a more static molecule and more easily forms the ES water clustering. Therefore D2O can accommodate the guest molecules more easily without breaking its hydrogen bonds [874]. The addition of positively hydrating salts (for example, LiCl) that destroy the water low-density ES clustering reduces the solubility ('salt out') of the nonpolar gases. In contrast, hydrophobic hydrating salts (for example, tetramethylammonium chloride) that increase water low-density ES clustering stability also increase nonpolar gas solubility ('salt in'). Small nonpolar organic molecules also behave similarly to nonpolar gases, but their increased size alters the clathrate structuring. Thus benzene has a solubility minimum, at a lower temperature than expected from above, at about 20 °C [210].
Interestingly, the change in solubility of nonpolar gases with respect to their diameters has a maximum (and their free energy of hydration has a minimum) when diameters are about the same as that of the dodecahedral cavities (that is, ≈ 4.5 Å) in the icosahedral network [196]. The solubility behavior of larger hydrophobic molecules is discussed briefly elsewhere. It should also be noted that the solvent properties of superheated liquid water also change with temperature as water's dielectric permittivity reduces towards that of common organic solvents as the temperature rises towards its critical point. Even though the amount of air (that is, N2 + O2 + Ar) dissolved in water is very low, it is sufficient to lower the density of water by almost 5 ppm (that is, 0.0005%) at 0 °C [870].
It should be clear from the above discussion that the solubility of nonpolar gases (at equilibrium), in water at its point is not zero, an error propagated by some textbooks. However, the non-equilibrium production of steam would lead to the temporary loss of dissolved gas from the liquid phase, and this would increase the pressure in the closed system required for solubility determinations.
The solubility of gases (and other solutes such as salts) in ice is very low and this explains the usefulness of freeze-thaw operations under reduced pressure for degassing water.
[![]() Anomalies page: Back to Top
Anomalies page: Back to Top ![]() ]
]
The high relative permittivity (dielectric constant) of water and its small molecular size combine to endow water with the strong ability to dissolve salts. Polar molecules, where the centers of positive and
negative charges are separated, possess a dipole
moment. This means that in an applied electric field,
polar molecules tend to align themselves with the field. Although
water is a polar molecule, its hydrogen-bonded network tends
to oppose this alignment. The degree to which a substance
does this is called its dielectric constant (permittivity). Because water possesses a hydrogen-bonded network that transmits polarity shifts extensively through rapid and linked collective changes in the orientation of its hydrogen bonds, it has a high
relative permittivity (dielectric constant). This allows it to act as a solvent for
ionic compounds, where the attractive electric field between
the oppositely charged ions is reduced
by about 80-fold, allowing the thermal motion to separate
the ions amongst the solution. An ab initio molecular dynamics study showed that the increase of the average dipole moment (due to hydrogen-bonding) of water molecules and the local alignment of these molecular dipoles both contribute almost equally to this high relative permittivity (dielectric constant) [1672]. On cooling, as the water network strengthens and water's dipole moment increases, the dielectric of liquid water climbs to 87.9 (0 °C), increasing on conversion
to ice to 96 (0 °C) then rising further to about 183 as the ice is cooled to 140 K. The increase in relative permittivity on freezing is anomalous as the relative permittivities of most polar liquids fall abruptly on freezing (e.g., nitrobenzene 37 ![]() 3) to close to the square of the refractive index due to molecular rotations ceasing. The freezing of water is different due to the relative permittivity being mainly due to proton hopping rather than molecular rotation.
3) to close to the square of the refractive index due to molecular rotations ceasing. The freezing of water is different due to the relative permittivity being mainly due to proton hopping rather than molecular rotation.
The relative permittivity (dielectric constant) drops on heating, and liquid water becomes far less polar, down to a value of about six at the critical point. The relative permittivity (dielectric constant) similarly reduces if the hydrogen bonding is broken by other means such as strong electric fields but not with pressure. The change in the dielectric with temperature gives rise to considerable and anomalous changes in its solubilization and partition properties with temperature. These are particularly noticeable in superheated water [610], where the dielectric is low, and in supercooled water high dielectric. Also, the dielectric increases (107.7 at -35 °C, with a sudden jump to >10,000 at -40 °C in nano-sized pores [1774] ) even as the density decreases. Pressure increases the relative permittivity (dielectric constant) (101.42 at 0 °C and 500 MPa), due to its effect on the density.
Perhaps the high relative permittivity (dielectric constant) of water should not be considered anomalous as other small polar molecules (with higher dipole moments) form liquids also having high dielectric constants (see below). The ratio dielectric constant/(dipole moment)2 is often also reckoned, by others, to be anomalously high in liquid water (but note that the gas-phase, rather than liquid, dipole moments are used for comparing these substances). Although high, clearly molecules with zero dipole moment (e.g., CCl4) have infinite such values. Also anomalous is the 60% increase in the static dipole moment of water molecules in liquid water compared to the gaseous state. This is due to hydrogen bonding causing greater intermolecular charge transfers during the changed distance between the molecular charge centers. Interestingly, the dynamical dipole moment n is not similarly enhanced and may even be reduced due to the electrostatic dragging effect of surrounding electrons [1993].
Dipole moments and dielectric constants

Shown opposite are the dipole moments (blue triangles below) and dielectric constant/(dipole moment)2 ratios (red diamonds above) relative to the dielectric constants for a range of solvents. The data 1-17 correspond to 1, diethyl ether; 2, chloroform; 3, methylene dichloride; 4, methyl ethyl ketone; 5, acetone; 6, ethanol; 7, methanol; 8, acetonitrile; 9, ethylene glycol; 10, dimethyl sulfoxide (DMSO); 11, hydrazine; 12, formic acid; 13, water; 14, sulfuric acid; 15, formamide; 16, hydrogen cyanide; and 17, N-methyl formamide respectively.
By measuring electrostatic forces, water shows an anomalously low dielectric constant (≈ 2) next to boron nitride (BN) surfaces [3319]. The depth of this (out-of-plane dielectric constant ≈ 2) layer is about 0.75 nm (3 water layers); it remains anomalously small (out-of-plane dielectric constant < 20) up to 20 nm and does not return to its bulk value (dielectric constant ≈ 80) for ≈ 500 nm. The rationale for this behavior is that the rotational freedom of water dipoles decreases near surfaces.
[![]() Anomalies page: Back to Top
Anomalies page: Back to Top ![]() ]
]
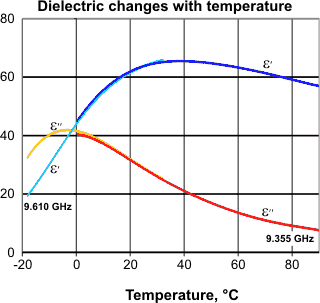
Anomalous dielectric behavior of water is found over a range of microwave frequencies between about 2 and 100 GHz . The real (ε') and the imaginary (ε'') part of the complex dielectric constant increase then decrease with increasing temperature. Examples at two close frequencies for liquid (including supercooled) water are shown opposite [588]. This may be understood by noting the shifts with the temperature of the maximum frequency of microwave absorption and the dielectric permittivity. Analysis of the complex permittivity gives a discontinuity at about 30 °C [1045].
The isopermittivity point, from [1698b]
![The isopermittivity point, from [1698] The isopermittivity point, from [1698]](images/isoperm.gif)
There is a frequency (2.96 ± 0.17 kHz.) where the relative permittivity (strictly speaking, the dielectric constant is the relative permittivity of a material for a frequency of zero) is independent of temperature (the isopermittive point). Below this frequency, the relative permittivity increases with temperature; above, it decreases. This has been explained by considering water as a system of two species: ions and dipoles [1698].
[![]() Anomalies page: Back to Top
Anomalies page: Back to Top ![]() ]
]
Dielectric constant and its pressure coefficient

The relative permittivity (dielectric constant) increases considerably at low temperatures. If carefully drawn, there appears to be a kink in the behavior at about 60 °C. This anomaly was associated with a subtle change in the water's average effective dipole moment from 2.17 D to 1.87 D [2741, 2755].
This behavior may be associated with solute solvatochromic shifts at about 43 °C in the specific hydrogen-bonded donor acidity, the hydrogen-bonded acceptor basicity, and the general nonspecific polarizability and dipolarity, as determined using solvatochromic probes [2892].q The temperature for this anomaly has a pH dependency from ~35 °C at pH 3.5 to ~45 °C at pH 7 to 9 [3828].
There is also a change in the dielectric with pressure (pressure coefficient) that also shows an unusual behavior [1912] with a minimum at about 95 °C thought due to the two-state structuring of liquid water.
[![]() Anomalies page: Back to Top
Anomalies page: Back to Top ![]() ]
]
The dielectric constant at 33 MPa, [2391]
The real and imaginary parts of the dielectric constant can be obtained by measuring the capacitance and loss tangent across the ice (H2O) under pressure. They show a minimum in the imaginary part near 20 K, but no such effect in the real part (see right, 33 MPa, [2391]).]). There was no such minimum found with heavy water ice (D2O), apparently due to the deuterium atom's heavier mass and reduced vibrational amplitude.
Concerted quantum tunneling of protons within hexamers
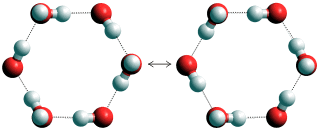
It is proposed [2391] that this anomaly is due to concerted quantum tunneling of protons within the water hexamers, similar to or as shown left.
The ionic mobilities of hydrogen ions and hydroxide ions at 361.9 and 206.5 (nm ˣ s−1)/(V ˣ m−1) at 25 °C are very high compared with values for other small ions such as lithium (40.1 (nm ˣ s−1)/(V m−1)) and fluoride (57.0 (nm ˣ s−1)/(V ˣ m−1)) ions. The Grotthuss mechanism explains this.
The limiting ionic conductivities are related (= mobility ˣ ionic charge ˣ Faraday) and their values for hydrogen ions and hydroxide ions, at 349.19 and 199.24 S ˣ cm2 ˣ mol−1 at 25 °C [737], are similarly very high compared with values for other small ions such as lithium (38.7 S ˣ cm2 ˣ mol−1) and fluoride (55.4 S ˣ cm2 ˣ mol−1) ions.
Another related anomaly is the preliminary report of an increase in both anion and cation mobilities at pH between 2.5 and 4.5 [1442].
Electrical conductivity and hydrogen ion concentration
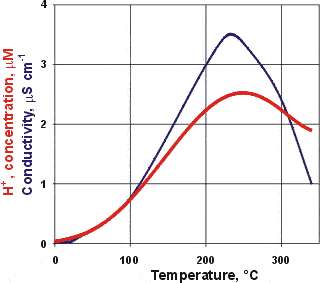
The electrolytic conductivity of water increases with temperature up to about 230 °C due mainly to its increased dissociation producing higher concentrations of the highly conducting H+ and OH− ions, which reach maximum concentrations at about 249 °C [IAPWS].
Above this temperature, for liquid water in equilibrium with the vapor, the density is much reduced (for example, 0.7 g cm−3 at 300 °C) reducing the ability for dissociation. Proton mobility decreases above 149 °C due to lowered amounts of 'Zundel' cations (that is, H5O2+) [1061].
Note that the pKw also reaches a maximum value at about 249 °C in line with the hydrogen ion concentration [IAPWS].
Salt impurities have a ten times percentage greater effect on conductivity at low temperatures than at higher temperatures (1 ppb NaCl gives a 1% increase at 85 °C but a 10 % increase at 0 °C) [737].
The resistivity of water, from [737]
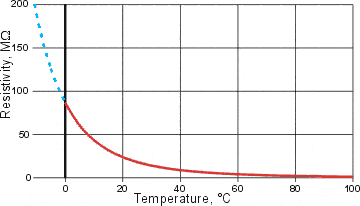
The significant increase in the water's resistivity (= 1/conductivity) at low temperatures [737] is shown left; extrapolated values are shown in dashed blue. Interestingly, the electrical conductivity of water increases on degassing [711]. Together these properties support the formation of ES clusters at low temperatures and in the presence of nonpolar gases, which involve localized and limited isotropic hydrogen-bonding and so prevent lengthy directed proton movements.
Conductivity at 0 °C, from [1984, 2171, 3571]
![Conductivity varying with electrolytic frequency at 0 °C [1984, 2171, 3571] Conductivity varying with electrolytic frequency at 0 °C [1984, 2171, 3571]](images/conductivity_frequency.gif)
The conductivity discussed so far is the static (direct current) conductivity. However, the electrolytic conductivity changes considerably with the potential frequency, see left at 0 °C, with the high-frequency conductivity (1 THz) being extremely high, due to the ease with which the water ionizes to short-lived H2O-H+···OH− ion-pairs [2171]. The concentration of these short-lived H2O-H+···OH− ion-pairs is about 1024 L−1 (≈ 1 M; about one in every 17 molecules of H2O at any instant [2171]).
Surprisingly at 100 kHz, the conductivity of ice Ih (≈ 0.4 µS cm−1) is about 40x that of water at 0 °C [2171].
[![]() Anomalies page: Back to Top
Anomalies page: Back to Top ![]() ]
]
pKa changes for the second ionization of phosphoric acid
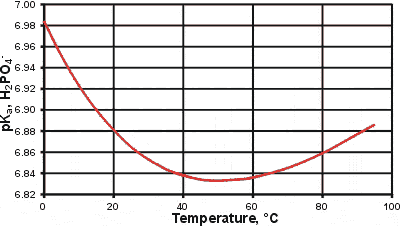
An interesting anomaly concerns the changes in the pKa with the temperature of many weak acids. As an example, we show this on the right for the second dissociation of phosphoric acid;
H2PO4− ![]() H+ + HPO42−
H+ + HPO42−
Such changes are due to a combination of factors, including changes in the dielectric (high temperatures increasing the pKa) and hydrogen-bonding (low temperatures increasing the pKa).
The unusually detailed X-ray diffraction is shown elsewhere and is simply explained by the presence of ordered clustering within the liquid phase. There also appears to be ordered structuring amongst the amorphous ices [3501].
[![]() Anomalies page: Back to Top
Anomalies page: Back to Top ![]() ]
]
Change in the nearest neighbor O-O distances, from [51]
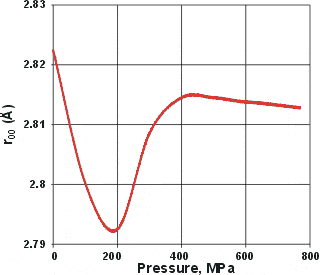
When liquid water is put under pressure (below about 200 MPa), the water molecules approach their neighbors more closely, as might be expected from the increase in density. However, if the pressure is increased from about 200 to 400 MPa, the average distance between neighboring water molecules increases [51]. This also occurs in supercritical water [3600]. At higher pressures, the distances reduce again (but less so) with increasing pressure. Similar and corroborative behavior is seen with the O-H stretch vibration frequency (v1), which increases with pressure between 200 to 400 MPa [533] while reducing with pressure at higher or lower pressures. The O-H stretch data has been confirmed at 23 °C but not found at 52 °C, indicating that it requires larger clusters to be recognized [824]
Cartoon showing how density increase can occur
without molecules getting closer (mouse over)
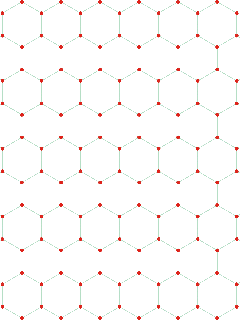
The explanation for all these effects is that there appears to be an increase in the interpenetration of hydrogen-bonded networks at about 200 MPa (at 290 K); interpenetration of hydrogen-bonded clusters being preferred over more extreme bending or breaking of the hydrogen bonds (see cartoon left where interpenetration increases the density without reducing the intermolecular distances. Such behavior can also be seen to cause other anomalies (1, 2, 3, 4, 5 ). When liquid water is put under pressure (below about 200 MPa) the water molecules approach their neighbors more closely, as expected from the increase in density. The interpenetration of the water networks gives rise to high-density water similar to the interpenetrating high-density ices.
The double pressure-derivative of κT, from [2194]
![The double pressure-derivative of kappa;T for pure liquid H2O at 273 C, [2194] The double pressure-derivative of kappa;T for pure liquid H2O at 273 C, [2194]](images/comp2d.gif)
The 200 MPa pressure required for the formation of high-density water has also been confirmed by infrared spectroscopy [2099]. Similar boundaries have been found using the isothermal compressibility data (see left) [2194].
A similar effect is found in supercooled water, where the distance between the water molecules decreases [1489] as the density decreases as the supercooling temperature is lowered. For example, the volume of one kg of water at 4 °C is 1.000 L with the water molecules 0.282 nm apart, but at -6 °C, the volume is 1.001 L with the water molecules only 0.279 nm apart, and in ice at 0 °C, the volume of one kg is 1.091 L with the water molecules only 0.276 nm apart. This decrease in density (increase in volume) is primarily due to the reduction in nearest neighbors. Also similar is what happens in the high-density ices where, for example, ice-seven (with two inter-penetrating cubic ice lattices) under a pressure of over 2200 MPa (density 1.65 g cm−3) has an average O····O nearest neighbor distance about 3.5% greater than that in cubic ice (density 0.92 g cm−3 at 0.1 MPa). Thus the density of ice-seven is somewhat less than twice the density of cubic ice (i.e., 2 ˣ 0.92/(1.035)3 = 1.65 g ˣ cm−3). Also, neighboring water molecules in hexagonal ice lie closer than those in liquid water, but the ice density is less due to ice molecules having only four neighbors.
A similar anomaly is that the first coordination shell positions of the amorphous ices move further apart as pressure increases and the amorphous ice densities increase [3501].
Formation Pressure |
0.1 MPa |
70 MPa |
1.1 GPa |
rO-O
, Å |
2.750 |
2.780 |
2.803 |
Density, g ˣ cm−3 |
0.9435 |
1.1347 |
1.2616 |
The bond lengths in the tetrahedral glass GeO2 also behave similarly as they increase under increasing pressure from 5 GPa to ≈ 30 GPa when the coordination of the Ge atoms increases [1930].
[![]() Anomalies page: Back to Top
Anomalies page: Back to Top ![]() ]
]
Electrical resistance versus potential
showing negative differential resistance, from [2223]
![Electrical resistance versus potential showing negative differential resistance (NDR); [2223] Electrical resistance versus potential showing negative differential resistance (NDR); [2223]](images/negative_resistance.gif)
Water coats all hydrophilic surfaces open to ambient atmospheres that are not dried, and the first absorbent layer is generally held strongly. Significantly this layer will affect the properties of the surface, including their electrical properties, and can cause negative resistance [2223]. This effect is shown right for Sr3Co2Fe24O41 under moist air conditions; a) normal ohmic conduction; b) breakdown tunneling; c) negative differential resistance, where the current decreases as the potential increases; d) water absorption [2223]. The negative resistance is expected due to the electrolytic breakdown of the adsorbed water.
[![]() Anomalies page: Back to Top
Anomalies page: Back to Top ![]() ]
]
a D2O is toxic to many organisms at high levels (20%-100% D2O, where it affects many processes, including mitosis and membrane function). However, it is not generally considered harmful at much lower levels where it is used in human physiological research. It would take many days of drinking 2-3 liters a day of deuterated water for a person to be poisoned enough to die. By use of a desiccation-rehydration process, it has been shown that the anhydrobiotic nematode Panagrolaimus superbus is able to tolerate and proliferate in 99% D2O due to its possession of a wide water channel pore [4234].
There is some evidence to show that artificially reducing its natural abundance in water (0.03% w/w) may have positive effects on the health of organisms [424]. [Back]
b This method for reconciling the data works poorly at low temperatures [1049]. [Back]
c The reduced zero-point energy when switching D-atoms for H-atoms from free to hydrogen-bonded positions within water clusters has been shown due to the energetic consequences of lowering the bend and torsional bond energies, which are greater than the raising of the stretching bond energy [986]. [Back]
d Also contributing to this effect are the relative isotopic differences between the zero-point energies of the liquid and gaseous phases. Librational vibrations (due to hydrogen-bonding) release energy when the phase changes from liquid to gaseous (where they are absent), with H2O librations (being greater) releasing more energy and so increasing the volatility of H2O relative to D2O at lower temperatures. Opposite effects are apparent at higher temperatures where there is less hydrogen bonding. However, energy still needs to be supplied to provide for the increased zero-point vibrational energy of the stretch vibrations (the gaseous stretch vibrations being more energetic than those for the liquid phase) [992]. [Back]
e In many gaseous-solute solvent systems (for example, N2 in CCl4), the solubility increases with temperature increase. Although solubility decreases with temperature increase are encountered with some other solute-solvent combinations (for example, methane in n-heptane), the behavior is a more general property of water and deserves comment. [Back]
f There is evidence [157, 269, 274] that the first (clathrate) shell possesses stronger hydrogen-bonding which weakens the hydrogen-bonding out to the next shell. [Back]
g The formation of O=O···H-OH hydrogen bonds may be seen as the first stage in the natural low-level formation of oxygen redox products (for example, H2O2) in water. As the ratio of O2/N2 solubilities has a maximum at 290 K, there is an indication that partial clathrate cages may be responsible for the polarization that encourages the hydrogen-bond formation. [Back]
i Nuclear quantum effects concern the different energies of the vibrational states. The deuterium atom bonds (being about twice as heavy as the protium atom) vibrate with less amplitude and frequency. Nuclear quantum effects are seen particularly in differences in their zero-point energy, the vibrational energy remaining at close to absolute zero. [Back]
j The term 'ideal' indicates adherence to a particular equation; it does not indicate any more fundamental truth. [Back]
l Within LDA clusters, the equilibrium lies more to the left, with HOD molecules being somewhat excluded [1779]. [Back]
m D2O is highly hygroscopic, absorbing water vapor from the atmosphere and so decreasing in D2O and increasing in HDO if handled in open vessels. [Back]
n The dynamical dipole is the behavior of the dipole (μ) upon molecular rotation, ∂μ/∂Ωξ (ξ = x, y, z), where Ωξ is the molecular rotation coordinate around the ξ axis [1993]. [Back]
o The molar volumes of most liquids and solids exhibit a volume decrease upon substitution with a heavy isotope [2621]. [Back]
p Zero-point energy is energy that remains even if the material could be cooled to 0 K (absolute zero, 0 K = −273.15 °C). As the hydrogen atoms of the water molecule are light, their motion is relatively great, and the molecules retain significant vibrational energy even at absolute zero. This zero-point energy allows easier transfer of protons between molecules and, hence, water's anomalously high conductivity. [Back]
q The solutes involved are for the specific hydrogen-bonded donor acidity (o,o-di-tert-butylstilbazolium betaine/ o-tert-butyl-stilbazolium betaine), the hydrogen-bonded acceptor basicity (5-nitroindoline/ 1-methyl -5-nitroindoline) and the general nonspecific polarizability (tetra-tert-butylnonaene ttbP9) and dipolarity (N,N-dimethyl-amino-7-nitrofluorene/fluoro-7-nitrofluorene). [Back]
![]() Phase anomalies (P1-P13) explanations
Phase anomalies (P1-P13) explanations
![]() Density anomalies (D1-D22) explanations
Density anomalies (D1-D22) explanations
![]() Thermodynamic anomalies (T1-T11) explanations
Thermodynamic anomalies (T1-T11) explanations
![]() Physical anomalies (F1-F10) explanations
Physical anomalies (F1-F10) explanations
Home | Site Index | The anomalies of water | Water: Introduction | The icosahedral water clusters | LSBU | Top
This page was established in 2006 and last updated by Martin Chaplin on 30 May, 2022